我们目前关心的记忆系统限定为两类时间记忆系统,系统随时间的演化不仅依赖于当前状态,也依赖过去的状态,一类是用时滞微分方程(组)描述的,其中的记忆体现在系统演化对当前时刻之前有限个过去状态的依赖性,另一类用分数阶微分方程(组)描述的,其中的记忆体现在系统演化对当前时刻之前所有过去状态的一种特殊的时间积累效应。作为时滞系统的重要应用之一,我们研究人体平衡系统的时滞与非线性动力学。
Here Dynamical systems with memory are restricted to two types of systems whose evolution with respect to time depends not only on the current state but also on the past states, the first type is described by differential equations with a finite number of discrete delays, and the other type is described by differential equations with fractional derivatives where the memory is a special integrated effect of all past states. As an important application, human balancing is studied with the response time considered.
❶
时滞反馈控制及时滞系统动力学
Dynamics of Systems with Delayed Feedback
时滞系统是一类无穷维系统,在振动控制与运动控制中,时滞主要来自控制器、滤波器和作动器的时延。时滞系统具有很多与无时滞系统本质不同的动力学特征,例如,一阶自治时滞微分方程即可发生Hopf分岔等多种非线性分岔以及混沌等非线性动力学现象。以振动控制、运动控制为背景,研究时滞反馈控制的参数整定、时滞系统稳定性判据和算法以及非线性动力学,发掘时滞系统的特有性质和特有动力学现象,发展非典型分岔条件下时滞系统非线性动力学的分析方法,结合系统动力学设计反馈控制策略。
Time-delay systems are infinite-dimensional. In vibration control and motion control, time delays are mainly from the time lag in controllers, filters and actuators. Time-delay systems are essentially different from delay-free systems in many aspects of system dynamics, such as Hopf bifurcation and chaos can be observed in some first-order autonomous delay differential equations. In view of vibration control and motion control problems, our focus includes parameter tuning of delayed feedback, stability criteria and testing algorithms, methods for nonlinear analysis under non-classic bifurcation conditions, control design on the basis of system dynamics.
❷
人体平衡系统的时滞与非线性动力学
Nonlinear and Delayed Dynamics in Human Balancing
人体姿态平衡是健康人体直立或行走的自然要求,考虑神经系统传输和反应时间的人体平衡系统是典型的时滞系统。在全球老龄化背景下,以减轻或避免摔倒伤害为目的,研究人体平衡系统的建模,控制策略,参数识别以及非线性动力学,探索人体平衡的生物力学机理,开发机器人辅助平衡装置。
Posture balance is a natural requirement for a healthy human being to stand upright or walk. Considering transmission and reaction time of nervous systems, a human balancing system is a typical time-delay system. In the context of global aging, our focus includes modeling, control strategy, parameter identification and nonlinear dynamics of human balancing systems, so as to explore bio-mechanism of human balancing and develop robotic-assisted balancing devices for the purpose of reducing or avoiding falling injuries.
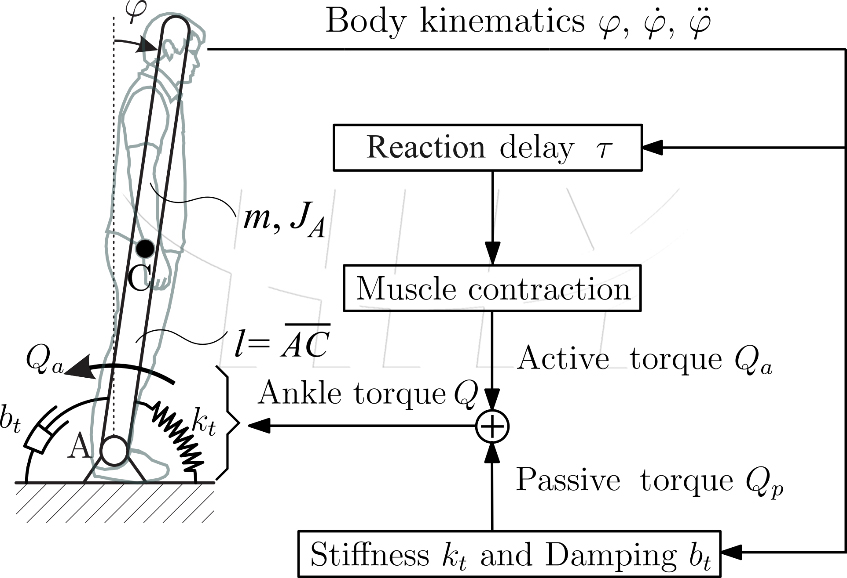
图1 矢状面内神经-力学人体平衡模型
Figure 1 Neural-mechanical model of balancing in sagittal plane
❸
分数阶系统的建模、分数阶控制与非线性动力学
Modeling, Control and Nonlinear Dynamics of Dynamical Systems with Fractional Derivatives
分数阶导数是整数阶导数的推广,它被认为是刻画记忆、描述中间过程或非局部特征的恰当数学工具。从振动的主被动控制角度看,有两种方式可以引入分数阶导数,一种方式是粘弹性材料作为被动阻尼材料,其特性介于理想弹性固体和理想流体中间,故粘弹性材料的本构方程可用分数阶导数来描述。另一种方式是在主动控制中引入分数阶导数,可大大增加控制参数的调节范围。以振动控制、运动控制为背景,研究分数阶系统的建模、稳定性判据和算法以及非线性动力学,分数阶微分方程的高效数值计算方法,发掘分数阶系统的特有性质和特有动力学现象,完善分数阶微分方程的基本理论。
Fractional derivative is a generalization of integer-order derivatives, and it has been proved an effective mathematical tool for describing phenomena with memory, intermediate process and non-local properties. In vibration control, fractional derivative is introduced in two ways, the first one is in the constitutive equation of viscoelastic materials as damping for passive control, and the other is the use of fractional-order controller that enlarges the parameter domain of active control. In view of vibration control and motion control problems, our focus includes modeling with fractional derivative, stability criteria and testing algorithms, numerical methods for solving differential equations with fractional derivatives, as well as improvement/correction of fundamental theory of fractional differential equations.





